The Behance Dot Grid Book is a coil-ringed notebook. It uses very thick paper (80 lb.), and is neither blank nor ruled – it has a “dot grid”.

Though very thick, the paper also seems pulpy and unfinished. I am wondering why this particular paper was chosen. Thicker paper being better was generally true with typewriters – but not necessarily with handheld writing and drawing implements.
The packaged notebook presents nicely (and for the price being asked for a 50 sheet coil-ring notebook, it has to) and includes an interesting brochure, “Make ideas happen.”

So why the dots? The band around the notebook states: “The geometric dot matrix on the front and back of each page serves as a subtle guide for your notations and sketches. The dot matrix pushes your ideas forward, beyond the confines of restrictive lines and boxes.” Really? It will push my ideas forward? More than a blank page? And no one finds dots constrictive?
Well, I’m mainly kidding. I think it’s great that they are exploring alternate “anchors” for paper writing. I recall seeing circular graph paper and all sorts of patterns in notebooks years ago, and am glad to have more choices.

After opening and contemplating this notebook in the backyard, I happened to glance at Mathematical Gems I by Ross Honsberger – part of the Dolciani Mathematical Expositions series. Chapter 11, “Circles, Squares, and Lattice Points”, tells us a great deal about the dots this notebook uses, which are formally called lattice points.

A very interesting problem is this: Can you draw a circle around just one dot? Trivial. How about two? Again pretty easy. Three? The problem just got a lot harder – but yes you can. So how about any arbitrary integer n? Amazingly, it is quickly proven that such a circle exists. And that’s just the beginning – Schinzel’s Theorem proves one can draw a circle with any given number of lattice points on the circle’s circumference – and Browkin’s Theorem proves that one can draw a square containing an arbitrary number of lattice points.
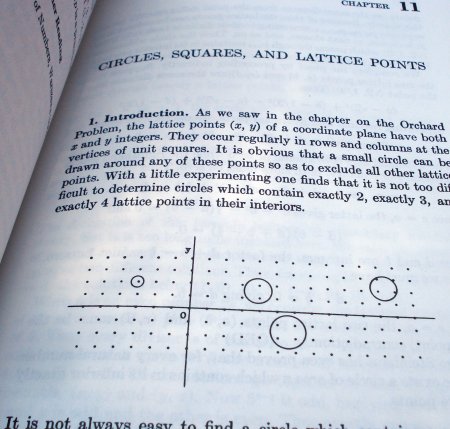
So if you want to play around and learn about some of these properties of lattice points, what better tool than the Behance Dot Grid Book?